Unit 1 Worksheet 3 Square and Square Roots class- 8 DAV Secondary Mathematics Solutions

Are you looking for DAV Maths Solutions for class 8 then you are in right place, we have discussed the solution of the Secondary Mathematics book which is followed in all DAV School. Solutions are given below with proper Explanation please bookmark our website for the further update !! All the Best !!

DAV SOLUTIONS CLASS 8 Secondary Mathematics Unit 1 Worksheet 3

Q1.Find the Square root of the following by prime factorisation.

i). 225 ii). 441 iii). 529 iv). 40000 v). 7744 vi).8281 vii).4096 viii).28900 ix).47089 x).24336

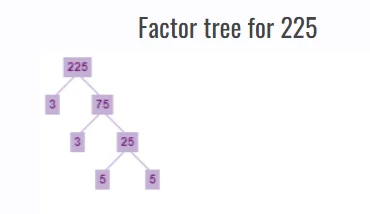
i). Prime Factorisation of 225 prime factors of 225 are 5 × 5 × 3 × 3.

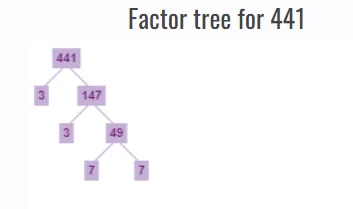
ii) Prime Factorisation for 441 the prime factors of 441 are 3, 3, 7, 7

iii) Prime Factorisation for 529 There are total 3 factors of 529 i.e. 1, 23, 529.

iv) Prime Factorisation for 40000 The prime factors are: 2 x 2 x 2 x 2 x 2 x 2 x 5 x 5 x 5 x 5

v) Prime Factorisation for 7744 The prime factorization of 7744 = 2⁶•11². See its prime factors tree below.

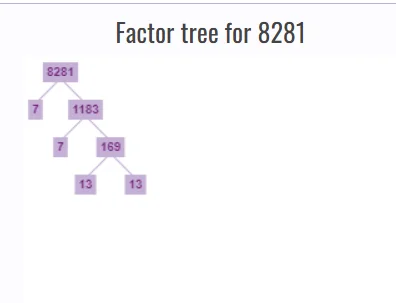
vi) Prime Factorisation for 8281 The prime factorization of 8281 = 7²•13². See its prime factors tree below.

vii) Prime Factorisation for 4096 The prime factorization of 4096 = 2¹². See its prime factors tree below.

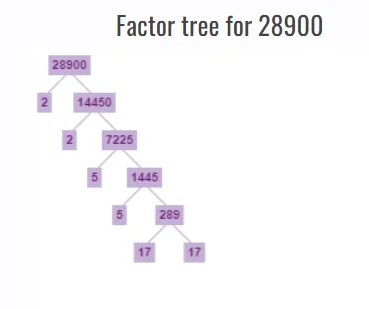
viii) Prime Factorisation for 28900 The prime factorization of 28900 = 2²•5²•17². See its prime factors tree below.

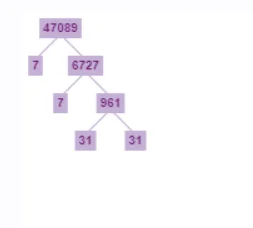
ix) Prime Factorisation for 47089 The prime factorization of 47089 = 7²•31². See its prime factors tree below.

x) Prime Factorisation for 24336 The prime factorization of 24336 = 2⁴•3²•13². See its prime factors tree below.

Q2. Find the smallest number by which 1100 must be multiplied so that the product becomes a perfect square. Also, find the square root of the perfect square so obtained.
Sol. Resolving 1100 into prime factors

1100 = 2 × 2 × 5 × 5 × 11
Here, 11 is unpaired. So 11 must be multiplied to 1100 to obtain a
perfect square number.
∴ The perfect square number so obtained
= 1100 × 11
= 12100

Q3. By what smallest number must 180 be multiplied so that it becomes a perfect square? Also find the square root of the number so obtained.
Sol. Resolving 180 into prime factors.
180 = 2 × 2 × 3 × 3 × 5

Here 5 is unpaired.
∴ 5 is required to multiply 180 to make it perfect square.
∴ Required number is 5 × 180 = 900
∴√900 = 2 × 2 × 3 × 3 × 5 × 5
= 2 × 3 × 5 = 30

Q4. Find the smallest number by which 3645 must be divided so that it becomes a perfect square. Also, find the square root of the resulting number.
Sol. Resolving 3645 into prime factors.

3645 = 3 × 3 × 3 × 3 × 3 × 3 × 5
Here 5 is unpaired. So the given number 3645 must be divided by 5 to
obtain a perfect square.
So the required number = 3645 ÷ 5 = 729
∴ √729 = 3 × 3 × 3 × 3 × 3 × 3
= 3 × 3 × 3
= 27

Q5. A gardener planted 1521 trees in rows such that the number of rows was equal to the number of plants in each row. Find the number of rows.
Sol. Let the number of rows be x.
∴ number of plants in each row = x
Total number of plants = x × x = x2
∴ x2 = 1521
⇒ x = 1521
⇒ √1521 = 3 × 3 × 13 ×13
⇒ √1521 = 3 × 13 = 39
Hence the number of rows is 39.

Q6. An officer wants to arrange 202500 cadets in the form of a square. How many cadets were there in each row?
Sol. Let there be x number of cadets in each row.
∴ Number of rows = x
Total number of cadets = x × x = x2
∴ x2 = 202500
x = 202500
√202500 = 2 × 2 × 3 × 3 × 3 × 3 × 5 × 5 × 5 × 5
∴ √202500 = 2 × 3 × 3 × 5 × 5
= 450

Q7. The area of a square field is 5184 m2. A rectangular field whose length is twice its breadth, has its perimeter equal to the perimeter of the square field. Find the area of the
rectangular field.

Sol. The area of the square field = 5184 m2
∴ side of the square = 5184 m
Resolving 5184 into prime factors
5184 = 2 × 2 × 2 × 2 × 2 × 2 × 3 × 3 × 3 × 3
∴ 5184 = 2 × 2 × 2 × 3 × 3 = 72
∴ Perimeter of the square field = 4 × side
= 4 × 72 = 288 m
Now, let the breadth of the rectangular field be x m.
∴ Length = 2x m
Perimeter of the rectangular field
= 2 [l + b] = 2 [2x + x] = 6x m
∴ 6x = 288 ⇒ x =
288
6
⇒ x = 48 m
∴ breadth = 48 m
and length = 2 × 48 = 96 m
∴ Area of the rectangular field = l × b = 96 × 48 = 4608 m2
Hence the required area = 4608 m2